In tennis, how much is the server's advantage diminished if the game goes to "deuce?"

Question

In tennis, the person with the serve has a clear advantage. This is is certainly true at love-love, where four points are needed to win a game.

A server who lets the game go to "deuce" has squandered part of that advantage. From that point, two (consecutive) points are needed to win.

What do tournament (or other) statistics have to say about the server's advantage from "deuce" as opposed to from love?"

And do the statistics say that the receiver is actually favored to win the set, starting from an "ad" (advantage)? From this point, the receiver need to win only one game, while the server needs (at least) three.

Answer 1

You reference certain statistics in your question and sadly, these types of stats don't exist for professional tennis to my knowledge. It would be really awesome if they did, though because they could answer some interesting questions like that one you pose here.

Having played a lot of tennis myself and having watched hundreds of matches on television, I would say the following:

1.) When the game reaches deuce, the server has some additional pressure on them because their opponent has demonstrated the ability to win as many points from their serve as they have. It also adds an element of pressure mentally if the player starts having thoughts like "if I lose this next point it will be break point. Don't screw this up!"

2.) Typically a player that has more of their service games reach deuce has to save more break points, and from stats that do exist, I can tell you that in most matches, the player that has to save more break points usually loses the match.

3.) When the player serving is playing "from behind" (meaning their opponent served first in the current set being played) - there is more pressure on that player to hold serve because if their serve gets broken by their opponent and then their opponent holds serve again, they will be trailing by 3 games. e.g. Their opponent holds for 1-0 lead, they get broken to go down 0-2, then their opponent holds again to go up 3-0. So a single break of serve can lead to a 3 game deficit.

Hopefully this helps - let me know if you are looking for clarification on anything else.

Answer 2

Bill Tilden speaks about doing an analysis like this in Match Play and the Spin of the Ball. His conclusion then, surprisingly, was that any proficient tennis player is at least even money to win from 0-40.

Answer 3

See if this sheds any light on it...

As long as the odds of winning any point stays the same throughout a game, then it's just a math problem... and calculations\graphs can be made. Since the exact advantage of serving is unknown (and varies by location, weather, gender, etc), and to also allow relative player skill to be factored in, graphs are made to show the spectrum of possibilities. Then some data for actual serve advantage is considered to attempt to more directly answer your question...

The graph below shows how much a player's odds of winning would change from the start of the game to deuce:

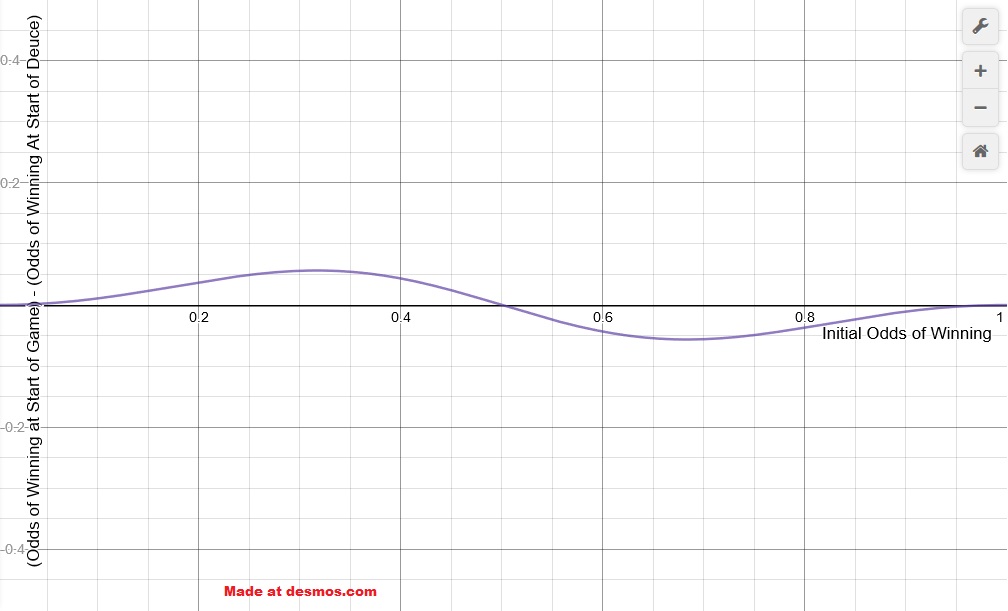
Look across for the player skill level. 0.6 = a player with a 60% chance of winning each point. His change is about -0.04, or a reduction of about 4% chance of winning.

The graph shows that the odds fall for a favored player, and go up for the inferior (or receiving) player. Makes sense. But it shows it's not really by a ton.

A more reasonable picture is really comparative odds...

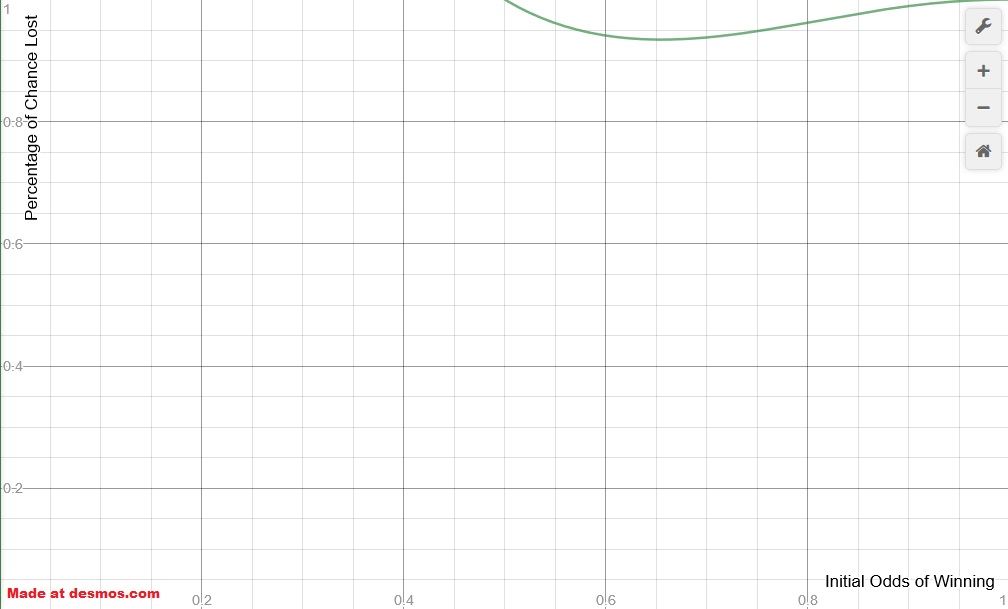
Similar idea. This one just shows what your odds are relative to where they started. So for a player that is 3 times better than his opponent (= a 75% initial chance of winning any point)... he would fall to just... 95% of his starting chance of winning the game if he is drawn out to deuce. His chance of winning was about 94.5% before starting, and is down to a 90% chance of winning when extended to deuce.

Worse case situation, a player is still 93% as likely to win a game as they were at the start. And the greatest absolute chance lost is only about a 6% chance of winning. The odds of winning don't change much.

I looked more for the estimated benefit of serving. Although the results of http://www.onlinetennisinstruction.com/win-more-with-one-statistic/ are perhaps shaky (Related Question), the information there from Wimbledon says men win somewhere in the 80-82% range of service games, and women win only 62-66%. So the odds drop to around 78% and 60% if the game extends to deuce. Still a very heavy majority for the server. [Note that the Wimbledon statistics still don't give the true serving advantage, since many Wimbledon matches are still unevenly matched players. But at least it allows us to say that in any random Wimbledon match, that would be the chance of the server winning a given service game. But unfortunately it can't just be combined with relative player strengths. Perhaps we could get a better estimate by looking only at matches that went to 5 sets, or between ones matching similar seeds!?!]

You can take a closer look at the graphs and equations used at https://www.desmos.com/calculator/ovvvauptv4 (as long as they are available). The green is the initial odds of winning a game, the blue is the new odds at deuce. The red is the change in odds. And the orange is the comparative chance. (I used 10 deuces, the change beyond that is minuscule)

Of course, this neglects things like psychological factors. It also excludes any impact from alternating ad and deuce courts. But I don't think either should have HUGE impacts. Would be great to compare to reality. But long story short, the result is: Win probability does not fall greatly when the game is extended to deuce.

---

To answer your second question, as to whether a returner with advantage becomes favored... a similar graph shows that a player would need at least a 35% chance of winning any given point to be favored to win the game after taking advantage. So based upon those Wimbledon numbers, a female that gets to a break point is slightly favored to win the game... but a male is still the slight underdog even at advantage.

(Being favored to win the break will always equate to being favored to win the set (and match) for any evenly matched players at any unbroken, even set score)

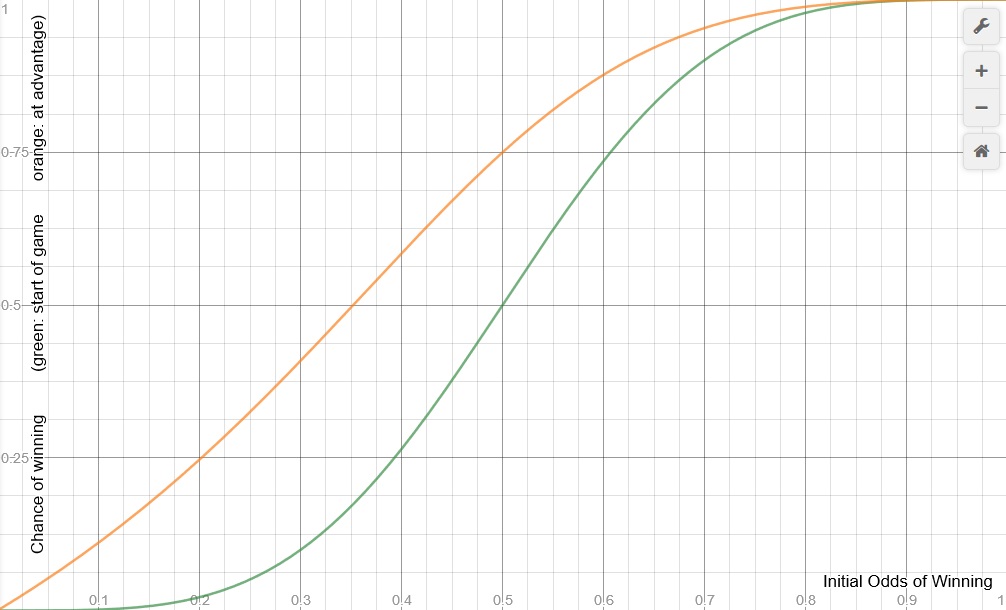
Hope that sheds some light on your questions. It's all theoretical statistics rather than match data, but it appears to be fairly concretely applicable.